The fundamentals of astronomy for beginners

EXPLAINER

---

Get to know your way around the celestial sphere

Jane Green explains the system that helps astronomers to navigate around the heavens

The early Greeks believed that stars were embedded in an enormous sphere rotating around Earth. Today we know that stars and all the other celestial objects lie at varying distances, and they only appear to move from east to west as a result of Earth itself spinning on its axis from west to east. However, in order to describe the positions and movements of stars above our horizon, astronomers still use this vast imagined shell. It is called the celestial sphere.

Projected onto this sphere is a grid system of reference points and lines, which are similar to maps used on Earth. Imagine Earth as a balloon with reference points and lines mapped on its surface; inflate the balloon until it fills the celestial sphere and Earth’s equator becomes the celestial equator, the celestial poles align with Earth’s rotational north and south poles, and the lines of longitude and latitude become celestial coordinates – respectively right ascension (RA) and declination (dec.) – found on star charts and planetarium software apps, such as Stellarium or Star Tracker, used by stargazers around the world.

Mapping the sky

Declination in the night sky is mapped in the same way that latitude is measured on Earth: in degrees, arcminutes and arcseconds north and south of the celestial equator. Measurements increase from 0° on the equator to 90° at the poles. Declination north of the celestial equator is detailed as positive (+), while south is negative (–). As examples, Polaris (Alpha (α) Ursae Minoris), the pole star for the Northern Hemisphere, has a declination of just over +89° and Rigel (Beta (β) Orionis), the blue supergiant star marking the Hunter’s foot in the constellation of Orion, is positioned at –8° 15 arcminutes below the celestial equator.

The celestial equivalent of Earth’s longitude is called right ascension (RA) and although it can be shown in degrees, it is normally expressed in units of time: hours, minutes and seconds.

Twenty-four hours equals 360° and one hour of RA equals 15° of arc. The RA of the star Polaris is therefore described as 2 hours, 59 minutes and the RA of Rigel is 5 hours and 15 minutes. Every object in the heavens can be located by using these RA and dec. coordinates.

The zero (0) line of RA – the celestial equivalent of Earth’s Greenwich Meridian of longitude – passes through the point where the Sun crosses the celestial equator at the first moment of spring in the Northern Hemisphere, the vernal equinox. Hours in RA are measured eastward from this point until 23 hours, 59 minutes is reached. One minute later and we return to 0 hours.

Dividing the celestial sphere is a line denoting the ecliptic, marking the apparent path of the Sun across the background stars – located by tracing a line between one or more planets and the Moon.

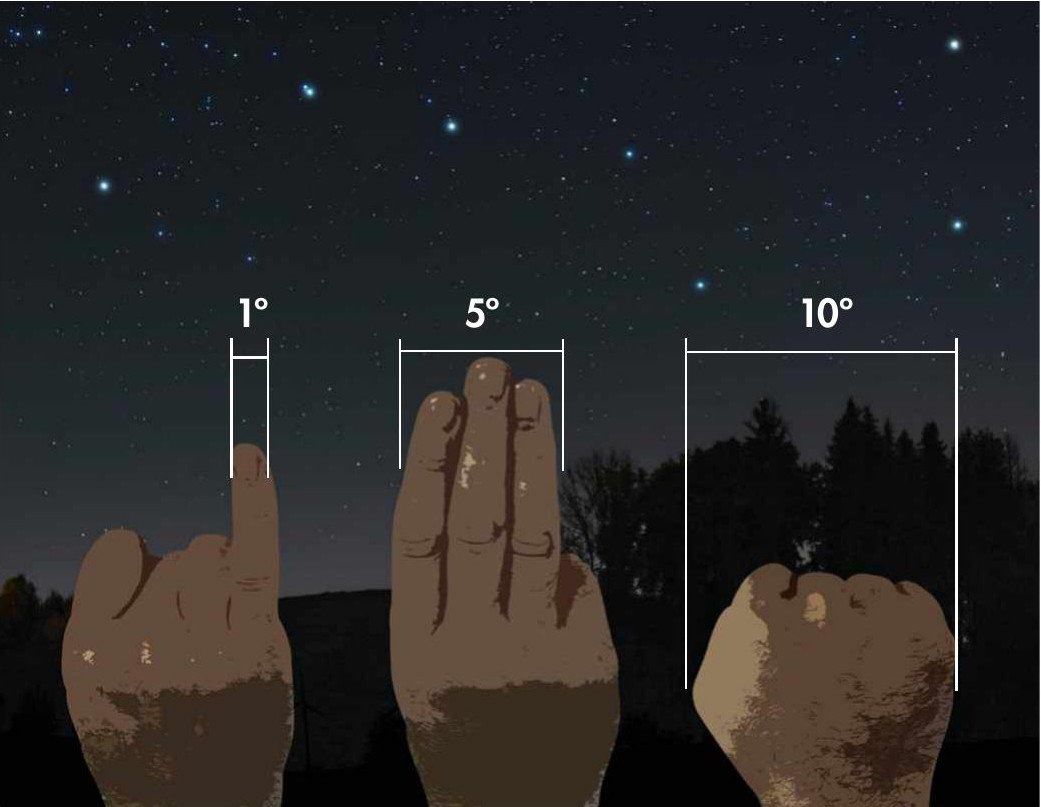
Which stars can be seen and how they appear to move with respect to your location can be conceptualised using the celestial sphere. At the North Pole (90°), the North Celestial Pole corresponds with the zenith – the point directly overhead. The celestial equator is now parallel with the horizon and as stars move along a path parallel to the horizon, only those in the northern half of the celestial sphere are visible. At Earth’s equator (0°) you can see all the stars since the celestial equator arcs from horizon to horizon via the zenith and the North and South Celestial Poles lie on the horizon. The stars rise straight up in the east and sink straight down in the west. Between these extremes, part of the sky always remains invisible. So if you’re observing at either pole the stars will circle overhead and never set – they are circumpolar – but the stars circling the opposite celestial pole will remain invisible. Your hands and fingers held at arm’s length are ideal measuring tools for navigating the celestial sphere; an outstretched hand measures around 22° – the width of the Plough – and your little finger equals 1°, the width of two full Moons.

Telescopes and star coordinates

How different mounts are used to guide telescopes using RA and dec. coordinates

Altaz mounts have two axes of rotation: one moves in elevation – up and down – 0° to 90° (altitude); and the other moves in a 360° circle parallel to the horizon (azimuth).

Equatorial mounts are like altaz mounts, but tilted at an angle based on the observer’s longitude, which corresponds with Polaris’s altitude. They compensate for Earth’s rotation by having one rotational axis – the RA or ‘polar’ axis – parallel with Earth’s axis of rotation. They are moved by manual adjustment using RA (right ascension) and dec. (declination) setting circles, or a small electric motor on the axes, within the RA and dec. coordinate system, which allows all-night tracking of objects on the celestial sphere.

Fully motorised and computerised Go-To mounts remove the need to know RA and dec. coordinates, as brighter objects are listed on a database accessed at the press of a button, but RA and dec. are still useful for locating fainter targets.

Photos: JANE GREEN/STELLARIUM, URSA MAJOR IMAGE: RASTAN/ISTOCK/GETTY IMAGES, PAUL WHITFIELD X 2